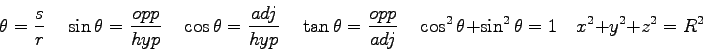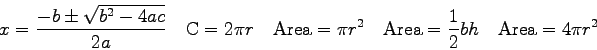
![\includegraphics[height=1.5in]{balls.eps}](img37.png)
I pledge that I have neither given nor received unauthorized assistance during the completion of this work.
Signature height0pt depth1pt width3in
Questions (9 pts. apiece) Answer in complete, well-written sentences WITHIN the spaces provided.



![\includegraphics[height=1.5in]{balls.eps}](img37.png)
Problems. Clearly show all reasoning for full credit. Use a separate sheet to show your work.
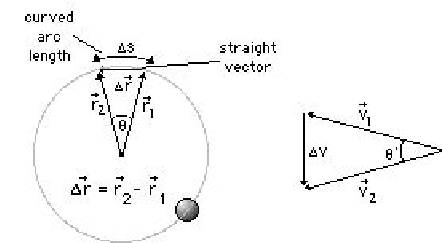
| 1. | 15 pts. | The astronaut orbiting the Earth in the figure is docking with the Westar VI satellite.
The satellite is in an orbit 650 km above the Earth's surface where the free-fall acceleration
is
|
| 2. | 20 pts. | A driver moving at |
| 3. | 20 pts. | During a volcanic eruption chunks of rock are blasted out of the volcano.
These projectiles are called volcanic bombs.
Consider the figure below of Mount Fuji in Japan.
A volcanic bomb is launched at an angle
![\includegraphics[height=2.0in]{MountFuji.ps}](img13.png)
|
| Speed of Light ( |
|
proton/neutron mass |
|
| Gravitation constant |
|
Earth's radius |
|
| Earth-Moon distance |
|
Electron mass |
|