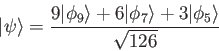
and a first measurement of the energy is made with the result
I pledge that I have given nor received unauthorized assistance during the completion of this work.
Signature:
Questions (6 pts. apiece) Answer questions in complete, well-written sentences WITHIN the spaces provided. For multiple-choice questions circle the correct answer.
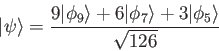
Problems. Clearly show all work for full credit.
| 1. (20 pts.) |
The number of hairs |
||||||
| 2. (20 pts.) |
The time-dependent Schroedinger equation in one dimension is the following.
|
||||||
| 3. (30 pts) |
Consider a case of one dimensional nuclear
`fusion'.
A neutron is in the potential well of a nucleus that we will
approximate with an infinite square well with
walls at 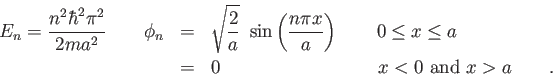 The neutron is in the
|
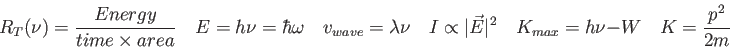


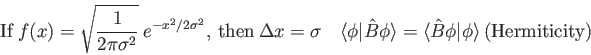

The wave function,
![]() , contains all we know of a system and
, contains all we know of a system and ![]() is the probability of finding it in
the region
is the probability of finding it in
the region ![]() to
to
![]() .
The wave function and its derivative are (1) finite, (2) continuous, and (3) single-valued.
.
The wave function and its derivative are (1) finite, (2) continuous, and (3) single-valued.
| Speed of light ( |
|
fermi ( |
|
| Boltzmann constant ( |
|
angstrom ( |
|
|
|
electron-volt ( |
|
|
| Planck constant ( |
|
MeV | |
|
|
GeV | ||
| Planck constant ( |
|
Electron charge ( |
|
|
|
|||
| Planck constant ( |
Electron mass ( |
|
|
|
|
|||
| Proton mass ( |
|
atomic mass unit ( |
|
| Neutron mass ( |
|
||